It only takes a group of 23 people for there to be a greater than 50% chance that two people share a birthday.

In any group of people there is a chance that at least two people share a birthday – but what are the chances? Surely it doesn’t take 366 people before two of them have the same birthday, but how many do you need? The Birthday Paradox (aka the Birthday Problem) is so named because it demonstrates our generally poor ability to intuitively reason more complex mathematics. Humans are bad at grasping big numbers, we’re bad at compounding, and as demonstrated in the birthday paradox we’re bad a probability.

Birthday Probability

Because of our poor ability to understand probability people may be surprised to learn that, despite 365 possible birthdays (excluding leap day), it only takes 23 people for there to be a 50.7% chance that two people have the same birthday (factoring in leap days it becomes a 50.6% chance). With 50 people it jumps up to 97%, and by 60 people a shared birthday is all but guaranteed at a 99.4% likelihood.

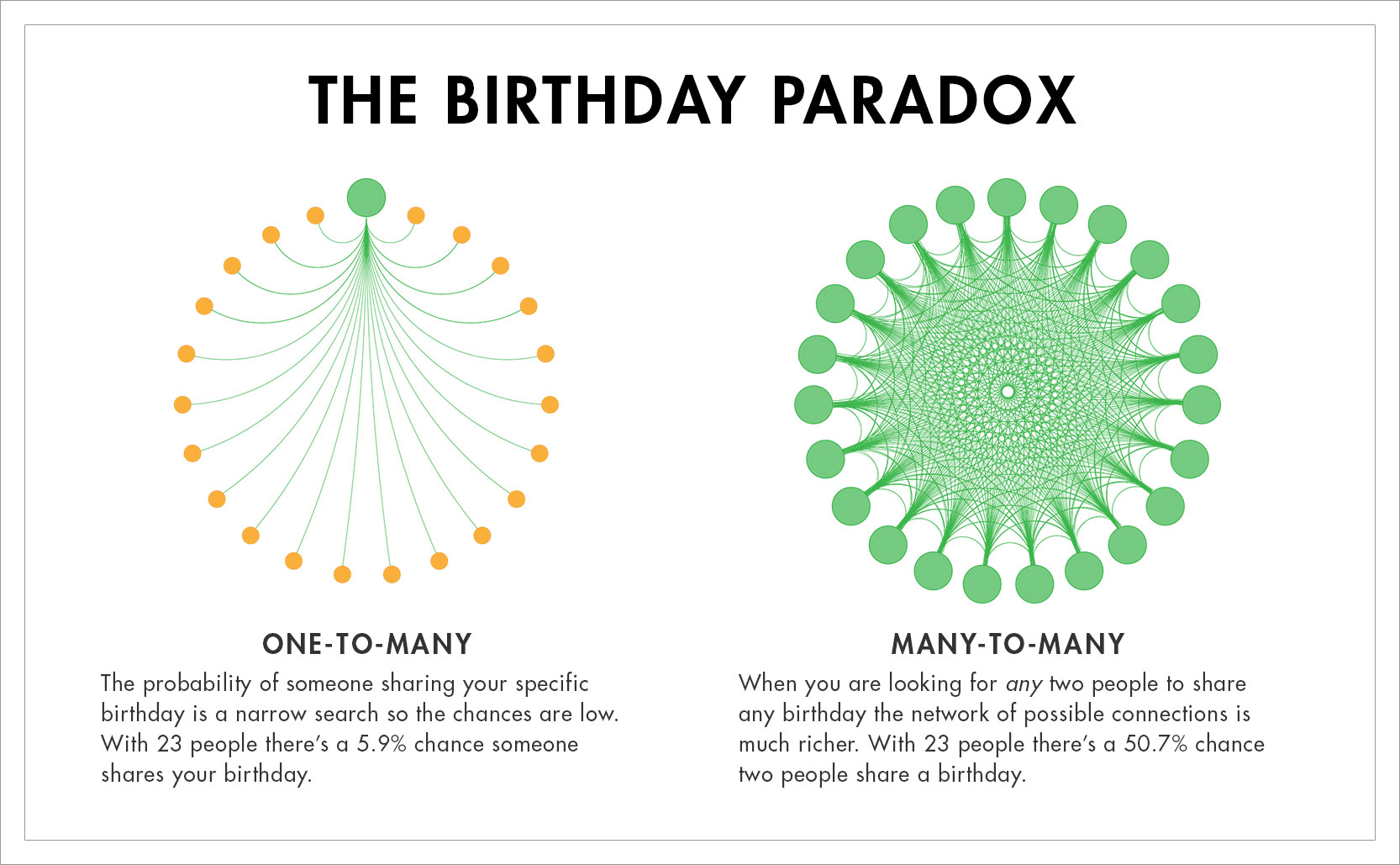
To explain why it takes so few people it’s important to consider how you approach the problem. If you are thinking of the odds that a single person has your birthday, it’s 1/365 or 0.27% chance (so not very likely). In a group of 23 people the odds that any of them have your birthday is 0.27% x 22 other people, so around 5.9% – still pretty unlikely. However, when you broaden the perspective away from yourself where 23 people are bringing 23 possible birthdays, creating 253 different birthday pairings, where any of them might overlap, now you’re pulling from a much larger probability pool. This many-to-many network of possibilities is how there can be a 50.7% chance that two people share a birthday.

Added info: There are a variety of online calculators that allow you to find the probability of sharing a birthday. You can find the likelihood of someone sharing your birthday as well as the likelihood of two people in general sharing a birthday.